In 1905, Einstein famously published four papers, each of which caused a revolution in physics. Although he is better known for his theory of relativity, it was his 1905 explanation of the photoelectric effect that won him a Nobel Prize.
In the photoelectric effect, UV radiation falling on the surface of a metal can make the metal emit electrons. This turned out to be difficult to explain based on the then-current theory of light as a wave. Experimenters had observed that the kinetic energy of the emitted electrons depends on the frequency of the radiation, not the intensity. The wave theory of light predicts that intensity will be the controlling variable. Moreover, the effect has a threshold frequency, below which no effect is seen.
Max Planck had already postulated that light also had a particle nature. Einstein applied this idea to explain the photoelectric effect, arguing that light consisted of packets of energy and that an electron absorbed all of it or none of it. We now call such a packet a quantum (Latin for "How much?".) We call a quantum of electromagnetic radiation a photon.
In the first essay in this series, I suggested that, given the role of atoms in energy quantisation, we should see quantisation as a structural (aka "emergent") property, rather than a substantive (aka fundamental) property. In this essay, I will pursue this argument a little further and then comment on the myth of quantised gravity.
What is and is not Quantised?
Quantisation is simply a fact of nature. Some quantities are naturally quantised. These include
- Franck-Hertz experiment (1914): Showed electrons in gases absorb energy in discrete steps.
- Stern-Gerlach (1922): Silver atoms deflect in discrete trajectories, proving spin quantization.
- Zeeman effect (1896): Magnetic fields split spectral lines into discrete frequencies.
- Neutron interferometry (1970s): Confirms half-integer spin for fermions.
- Millikan’s oil drop (1911): Measured charge strictly as ne.
- Quantum Hall effect (1980): Conductance steps at e2/h
However, we know of several quantities for which there is no evidence of quantisation.
- Space and Time: No experiment has ever detected quantized spacetime.
- Mass: There is no evidence of a discrete unit of mass. And again, no observation requires it.
- Gravitational waves show no signs of discreteness. No quantum theory of gravity successfully describes observation. And no explanation of an observation so far made requires quantum gravity.
- Fluids/Collective Phenomena: Sound waves, ocean waves, etc., are not discrete.
At present, it is still unfortunately the case that quantum theory has not offered us any coherent explanation of the atom or subatomic world. There is certainly no reason to believe that quantum physics explains anything at all about the world in its current state. It's merely a mathematical formalism that accurately predicts the probabilities of all outcomes for any observation.
Attempts to find the physical meaning of this have amounted to reifying the probability distribution. I will cover the metaphysics of probability in my next essay.
For now, we can simply note that no attempt to reify the wavefunction has resulted in a coherent metaphysics. We are left with a number of mutually exclusive metaphysical speculations that manage to "explain" quantum mechanics at the cost of having to abandon Realism and locality.
99% of physicists are apparently willing to pay this toll. While a dogged band of eccentric tenured professors (who can't be sacked for heresy) and a ragged collection of outsiders continue to try to do better and rescue Realism in the process. This essay, and the previous two, are my reflections on how we could do better.
Particles and Properties
In my view, the term "particle" is confusing. For physicists, it continues to conjure the idea of a point mass, which is to say a particle that has mass but no length dimensions. The problem with point masses is that density is mass divided by length. If an electron had finite mass but zero length (radius or volume), it would have infinite density since x/0 = ∞; for all values of x. That is to say, every electron would be a tiny blackhole. Observation tells us this is not the case. So we can simply, logically, rule out any talk of "point masses" or "point charges". They are mathematical fictions.
We often assume, for the purposes of calculation that all the mass of an object is concentrated at the centre of mass. This massively simplifies mechanics. But it is precisely in atomic physics that such idealised models run into trouble.
In our universe, there are no points, no singularities, and no infinities (though some quantities may be uncountable). The same observation means that general relativity is incomplete, since it currently predicts singularities in black holes and at the Big Bang. Indeed, at the Big Bang, all energy was supposedly contained within a tiny volume, creating near-infinite spacetime curvature. If the universe started out that way, then it was a black hole, and nothing ever escapes from a black hole. There are dozens of ideas about how to prevent singularities in GR, but as far as I can see, there is no consensus on the horizon.
Wave-particle duality made enough sense in the 1920s that no one seriously questioned it. In the 2020s, however, it has become clear that "particle-like" behaviour is an artefact of detector design and, as I have explain, related to the standing wave structure of the atom. Matter is waves (likely soliton waves in spacetime). However, this realisation did not lead professional physicists to go back to the original formulations and root out all mention of particles. Rather, wave-particle duality is still retained as a fundamental postulate even in quantum field theory.
To put it another way, one of the quantities that physicists still use the maths for is to find the "position" of the electron in an atom. And one way of talking about superposition is that the electron in an atom is in all possible locations at once (hence the idea of electron "clouds". But note that the mass of all these superpositions is still just the mass of the electron. I'm not sure how this can be explained. Can an electron in a superposition have fractional mass? When you ask physicists this is the kind of question that they seem to struggle. I assume that this is because the question is not canonical. The answer is not in the textbooks.
That said, the electron-as-wave has a very clear physical reality. The electron has mass, charge, and angular momentum. These are all real quantities that can be measured. Moreover, electron interact in causal ways. So the electron behaves like a real object with objective properties that can be measured precisely. So why the formalism is so vague I do not understand. But since "particle" is inaccurate and carries baggage, I propose to use the term wavicle for such objects.
I discussed the roles of substance and structure in reality at length in a series of three essays in 2016. In the meantime, I've decided to drop the terms "fundamental" and "emergent", which are legacies of metaphysical reductionism. Reductionism holds that only the irreducible (i.e. "fundamental") features of the world are real. In this view, "real" and "fundamental" are synonyms. The corollary is that structure is not real. I reject this notion.
I take structure to be every bit as real as substance. For example, structure can persist over time, we can interact with structures as objects (over and above any interaction with their parts), and structure is capable of participating in sequences of events that appear causal.
Note, I need to go back over the issue of causality. Since the last time I wrote about it, I've learned that causality is, in fact, built into relativity. Relativity takes as axiomatic the idea that causality is independent of frame of reference. Time and space are relative to one's frame of reference, but causality is not.
While reductionism is the optimal approach for studying substances, it gives us no leverage on understanding structures. Most of the significant properties of water, for example, are due to the arrangement of the three atoms that make up the molecule. For example, while both hydrogen and oxygen atoms are electrically neutral, the water molecule is an electric dipole. As such, electrostatic forces created by the dipole play a significant role in how water behaves. This is why, for example, water has such a high surface tension and a high specific heat compared to similar liquids.
In my view (influenced by Jones 2013), there are two kinds of properties.
- Substantial Properties are the result of substance. Substantial properties are unaffected by the imposition of structure (no "downward" causation).
- Structural Properties are the result of structure. Structural properties are (at least to some extent) independent of substance.
This is a static view. If we need to talk about a dynamic structure, it can be helpful to switch to the language of systems and speak of systemic properties. A "system" is simply a persistent dynamic structure. And a structure is a static system. Keep in mind that "static" and "dynamic" are idealisations. In the real world, things are always in motion, even those that persist over time.
How we perceive such systems is affected by the scale on which we observe them. On the millimetre scale, for example, few objects are as stable and unchanging as diamonds, which were formed in the mantle of the Earth millions of years ago. On the picometer scale, the diamond resolves into a vibrating lattice of vibrating atoms, with everything in constant motion. Matter is vibrant.
Whether we experience it as a static structure (a diamond) or a dynamic system (vibrating lattice) depends on scale. There's inevitably a transition zone in which making the distinction is difficult. We simply choose whichever viewpoint suits our aims.
That said, I think the substance/structure gestalt is helpful for anyone wishing to move beyond the limits of reductionism and into a more coherent view of reality. After all, almost everything that we can interact with is a structure or system. And our language about the world is based on how we physically interact with such objects.
Quantisation of Energy
In my first attempt at a description of the hydrogen atom, I described the electron as a (real) spherical standing wave (I leave open for the time being the question of what is waving, but I'm leaning towards the answer being "spacetime"). The basic idea is not new; both Heisenberg and Schrodinger started off with a similar approach. The difference is that they start off assuming wave-particle duality, which we now know to be a false step. Let's look again at the concept of a standing wave.
Standing Waves on a String
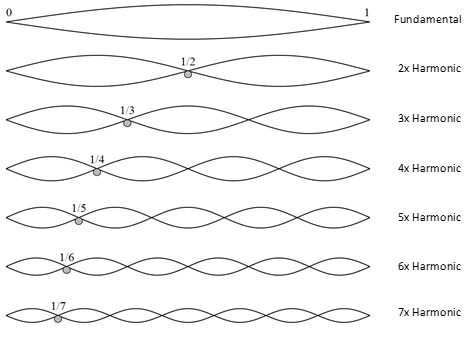
A guitar string is anchored at the ends by the "bridge" and the "nut". No matter how much a string is vibrating, the amplitude of vibration at the ends is always zero. We call these points nodes. If I tune a guitar string to a pitch and strike it, other things being equal, it will always vibrate at that precise pitch (pitch is determined by the density of the material, the radius of the string, temperature, and the tension it is under).
Note that because the ends of the string are anchored and cannot vibrate, a guitar string is only capable of vibrating in standing waves. Once you impose boundary conditions such as anchoring the ends, the oscillations can only be standing waves.
Standing waves form on a guitar string when we strike it. Striking the string causes it to be locally stretched out of shape and experience a restoring force so that the stretched part accelerates back towards the centre but overshoots. The restoring force always acts towards where the resting state would be. This perturbation travels along the string in both directions. When it reaches the bridge or nut, the perturbation is reflected back along the string in the opposite direction.
If you think about how wavelength is defined in terms of sine waves (crest to crest), you can see that nodes correspond to where a cycle of the sine wave crosses the x-axis, i.e. at 0, π, and 2π radians. Thus, other things being equal, the fundamental mode (= "ground state" for an electron) corresponds to a wavelength of twice the string length.
Other things being equal, the string can also vibrate at higher energies, with pitches corresponding to the string length (L) divided by a positive integer.
For example, I can make a guitar string vibrate in the first harmonic mode. This is done by lightly placing a finger on the string at the halfway point (the 12th fret) to prevent it vibrating there, and striking the string with the other hand. This forces the string to vibrate at twice the frequency, with zero amplitude at the ends and in the middle. Now there is a node (zero amplitude) in the middle of the string as well as at the ends, corresponding to a whole wavelength. If the wavelength is halved, the frequency doubles. In music, we call this first harmonic the octave (referencing the fact that there are seven unevenly spaced notes in diatonic scales).
One can make the string vibrate at L/3 by damping the oscillations at 1/3 of the string length. In the case of a guitar, this corresponds to having nodes over the 7th and 19th frets. The second harmonic has a frequency of 3/2 of the fundamental. In music terms, this is an octave and a fifth above the fundamental.
Note that for n > 3, the nodes are no longer precisely aligned to the frets because the spacing between them is based on an equal temperament scale, to allow the guitar to sound in tune across different keys.
Also, in real life, guitar strings vibrate in two dimensions, and/or the plane of vibration may precess around the axis of the string. Strings also vibrate in many modes at once, and, in practice, the first harmonic is often more prominent than the fundamental.
There are many videos online explaining this, so if my explanation is not clear to you, I recommend looking up "harmonics". This video gives you a decent brief intro plus some basic maths.
Note. Some people refer to the fundamental as the "first harmonic". As a musician, I think of harmonics as additional modes of vibration. So the "first harmonic" in my view is the octave.
Standing Waves in a Sphere
As noted, in order to balance the spherically symmetric electric field of the proton, the electron in a H atom must be configured as a sphere. Any other configuration leaves the electrostatic field unbalanced, meaning that the atom would not be electrically neutral. However, all atoms are electrically neutral.
Ergo, electrons in atoms are spherical with the nucleus at the centre of the sphere. The nuclear reaction of an electron and a proton to form a neutron (and a neutrino) is endothermic, requiring energy to make it happen. This means that it is not thermodynamically favoured despite the strong electromagnetic attraction. And this means that electrons seldom combine with protons except in places like the core of stars.
Keep in mind here that I'm describing an idealised situation in which no external forces are acting on the atom. In reality, everything influences everything else via the four forces.
Still, the electrostatic attraction between electron and proton is powerful and draws them together, pulling the spherical electron towards the centre. However, the electron wave has to accommodate a minimum number of wavelengths, so, despite the electrostatic attraction, it cannot get any smaller than the size needed to accommodate that number. Attracted inwards, the electron hits the minimum size limit, and it rebounds outwards. Just like the perturbation on a guitar string reflecting off the bridge and nut. The outgoing wave is prevented from escaping by the electrostatic attraction, which provides the restoring force. The outgoing wave stalls and accelerates back towards the centre again. With two waves of the same wavelength travelling in opposite directions, we have the conditions for the formation of a standing wave.
Energy radiates away from the guitar string in the form of sound waves and heat. And it gradually loses amplitude but stays at the same frequency. But there is no equivalent of this process in an atom, so the oscillation of the electron goes on indefinitely. Each atom is a kind of perpetual motion machine.
A logical consequence of this is that energy absorbed from or emitted by atoms will be quantised. The only way a resting state (fundamental) standing wave can absorb energy is for it to jump to a harmonic mode of vibration. The actual energies can be predicted from first principles using the maths of spherical standing waves developed by Pierre-Simon Laplace, ca 1782 (long before quantum mechanics was envisaged). Similarly, energy can only be emitted when an electron falls from a higher harmonic to a lower harmonic or to the fundamental.
For similar reasons, if the electron itself is emitted from a hydrogen atom, then it has a very specific energy corresponding to the ground state energy (looked at another way, this is the ionisation potential of the atom). An atom cannot capture an electron with less than the ground state energy. If it captures an electron with more than the ground state, then the excess will be emitted as a photon of that energy.
Outside of the atom, the energy of electrons is not quantised. A free electron can have any energy. Similarly, free photons can have any energy. We can design systems so that they emit photons of any arbitrary wavelength as long as the associated energy is greater than the ground state energy (ca. -13 eV). Any colour of light is possible, and for any two colours, we can always find an intermediate wavelength (even if the human eye can't resolve the difference).
However, note that when we need "free" electrons to study, we have to get them from atoms. So all the electrons we get to study always come pre-quantised. This may have blinded physicists to the role of the standing wave in energy quantisation and caused them to see quantisation as an independent idea.
This account explains the photoelectric effect and the ionisation energy of an atom as natural consequences of the standing wave configuration of the atom. And without invoking quantum mechanics.
Quantisation of "Spin"
A more complete treatment of the angular momenta of wavicles using a theory proposed by Jackson & Minkowski (2021) and similar to Macken's (2024) is in the pipeline. Today, I will just outline the basic idea. Spin is quantised for quite different reasons than energy. In this view, we think of the spherical electron as rotating. As I have shown, simple logic dictates that, in a hydrogen atom, an electron can only be a sphere. Any other configuration leads to unbalanced electrostatic forces and unstable atoms, because the electric field of the proton is spherically symmetrical.
It's less clear that a free electron has a shape, but we can account for at least some of its characteristics by modelling it as a tiny, rotating spherical soliton wave. This approach also comes with some non-trivial problems that I will address in a future essay.
We can illustrate the general principle using the planet Earth as an analogy.
Looked at from above the north pole, the Earth is rotating anticlockwise around the y-axis. Moreover, if I hovered above Cambridge at ~52° north, the Earth is still spinning anticlockwise. And so for any position that is not directly above the equator.
If I look at exactly the same planet from above the south pole, I see it rotating clockwise. Similarly, as I move from the pole (90° S) down towards the equator (0° S), I see the rotation as going clockwise all the way.
Now, if I measure the angular momentum of the Earth on the y-axis, from any angle, the vector will point along the y-axis in the positive direction (i.e. north). If it were spinning clockwise on the y-axis, the vector would point in the negative y-axis. And this is precisely analogous to "quantum spin" having just two values: "up" and "down" (with respect to the axis of measurement). If I measure the y-axis angular momentum of a sphere rotating on some (unknown) arbitrary axis, I will still see it as either pointing to +y or -y.
The analogy of rotation has been historically rejected for two main reasons. One of which was the strange behaviour of the angular momentum of spin ½ wavicles. If we impose a 360° rotation on a spin 1 wavicle, it behaves as expected: if we see it as spin up initially, following a 180° rotation we see it as spin down, and a further 180° rotation (360° in total), we see the spin as up again.
Spin ½ wavicles do not behave like this. If we rotate a spin ½ wavicle, such as an electron, it comes back to spin "up" in just 180° (though this state is not quite identical to the starting position). The hypothetical spin 2 wavicle only returns to spin up in 720°.
As a result, physicists generally abandoned the idea that angular momentum is related to rotation. Instead, they invented the idea of "intrinsic angular momentum", which doesn't involve any actual rotation. This is very dubious from a philosophical point of view. It's an ad hoc assumption that is only justified because it works mathematically, but it cannot be tested. And, in the process, it destroys any hope of a realist description of wavicles. Jackson and Minkowski have shown that we have to allow for gyroscopic effects of magnets in magnetic fields.
The gyroscopic effect is traditionally demonstrated in physics classes by an instructor sitting on a rotating chair holding a bicycle wheel. In this video for example, the wheel is pitching (rotating around the x-axis), the instructor attempts to make it roll (rotation around the z-axis), and the result is torque creating a yawing motion (rotation around the y-axis).
Something analogous seems to be happening to a spin ½ wavicles because they are magnetic dipoles (aka magnets). If a spherical magnet is rotating around the y-axis and I try to flip it around the z-axis using a magnetic field, then there is a torque around the x-axis. The result is that for a spin ½ wavicle that is rotating around the y-axis, a 180° rotation in the z-axis is accompanied by a 180° rotation in the x-axis. The direction of the angular momentum goes from up and back to up.
But it's not exactly the same, because the 180° rotation in the x-axis leaves the wavicle with reversed orientation in the z-axis. Another 180° rotation in z does bring it back to the starting position.
So for a sphere rotating about the y-axis, subjected to a 180° rotation about the z-axis:
- spin 0 wavicles cannot be rotated by magnetic fields.
- spin 1 involves a 180° rotation in z + 0° rotation in x.
- spin 2 involves a 180° rotation in z + 90° rotation in x.
- spin ½ involves a 180° rotation in z + 180° rotation in x.
This reproduces the observed properties of wavicle angular momentum without abandoning realism. And to date, spin 2 is purely hypothetical. Once again, there is a "classical" description of the system that predicts the behaviour we observe. And it is of a piece with the other classical physics that I have invoked in this series of essays.
The other, more serious problem with modelling the electron as a sphere is that, based on what was known a century ago, it was calculated that the surface speed of a point on such a sphere would be moving faster than the speed of light. There are ways around this, but it needs a lengthy explanation that I will work through in a forthcoming essay.
Conclusions and Gravity
To be clear, I'm not trying to explain (or justify) the postulates of quantum physics. Indeed, I think that postulates such as a wave-particle duality or fundamental quantisation are false. I am an old-fashioned kind of scientist, and I am only trying to explain observations. Philosophically, I remain committed to pragmatic Realism and the substance/structure gestalt (in place of reductionism).
As far as I can see, quantisation of energy (in experiments like the photoelectric effect) is driven by the structure of atoms, i.e. it results from standing waves, all of which are inherently quantised. It is the structure of the atom rather than the substance of the electron that imposes this configuration on matter. And the quantisation of angular momentum is because the spherical wavicle is rotating, with the proviso that spin ½ wavicles have a magnetic moment (they are magnets), so that using a magnet to rotate the angular momentum in one direction causes an orthogonal torque. This means that we can say:
Quantisation of energy is a structural property of atoms, rather than a substantial property of wavicles.
Or, for those who prefer the less precise legacy terminology:
Quantisation is an emergent property of atoms, rather than a fundamental property of particles.
And none of this is, in any way, "weird". Rather, we fully expect this behaviour from what we know about the (classical) mechanics of standing waves and spinning magnets.
An important corollary of this is that, where there is no standing wave involved, we should not expect energy to be quantized because there is no reason for it to be quantised. Where a wavicle is not a rotating sphere, we don't expect angular momentum to be quantised either.
A case in point is the gravitational wave. We have been measuring gravitational waves using the LIGO apparatus for a few years now. Gravitational waves are unequivocally real. The waves we detect are mostly from binaries of black holes or neutron stars colliding. It seems clear that such systems are not stable, and that (therefore) they do not form standing waves. Ergo, we would not expect gravity waves to be quantised.
Despite a century of strenuous efforts by a cadre of maths geniuses, there is still no way to meaningfully quantify gravity using the standard methods of quantum physics. The attempts to quantise gravity have not worked. And we should not expect this to ever work.
The quest for quantised gravity is not driven by any practical consideration or observation. Quantum gravity is not required to explain any existing experimental observations. Rather, the search for quantum gravity is being driven by ideological and aesthetic concerns.
Worse, physicists appear to believe that GR and QM ought to be reconcilable while at the same time acknowledging that neither theory is yet complete. My thought is that we cannot expect two incomplete theories to be reconcilable anyway. In all likelihood, completing them will solve the apparent problems.
Atoms, protons, and electrons are real. They persist over time and have measurable properties such as mass, charge, and angular momentum. In sequences of events, these entities play a causal role. In which case, I cannot see the logic in modelling atoms, protons, or electrons in terms of abstract probabilities. I believe this category error explains why the metaphysics that scientists have drawn from the formalism are so divergent and mutually exclusive: they are not anchored in reality.
The title of my next essay will be: "Ψ-ontologies and the Nature of Probability."
~~Φ~~
Bibliography
Jackson, Peter A. & Minkowski, John S. (2021). "The Measurement Problem, an Ontological Solution." SpringerNature, Foundations of Physics 51 (article 77).
———. (2014). "Quasi-classical Entanglement, Superposition and Bell Inequalities." Unpublished essay. https://www.academia.edu/9216615/
Jones, Richard H. (2013). Analysis & the Fullness of Reality: An Introduction to Reductionism & Emergence. Jackson Square Books.
Macken, John. (2024). "Oscillating Spacetime: The Foundation of the Universe". Journal of Modern Physics 15 (8): 1097-1143. DOI: 10.4236/jmp.2024.158047